Gravity

Imagine the universe is filled with water. Instead of empty space, every inch of it contains pure water. No planets, no stars, only water. What happens? And what would happen if an air bubble formed?

The answer to this question requires a basic understanding of gravity.


Gravity is very important. It helps hold matter together, bends light, and distorts space-time (which, incidentally, is how it bends light). It also makes it possible to play football, and as Americans are big football fans, they would certainly agree that we couldn't live without it.

Unfortunately, many Americans don't understand how gravity works. Admittedly, scientists haven't figured out a lot of things, but we do understand it well enough to make predictions and model physical events.

One of the important things about gravity is that its strength is proportional to the inverse of the square of the distance. In other words, it gets weaker as you get farther away, based on the equation:

F = c/d²

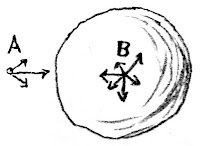
where c is a constant. So something that is twice as far away from Earth's center will weigh one quarter as much. Have a look at the diagram to the right to see an illustration of how this works. At twice the distance, gravity is spread out over four times the area.

Scientists didn't always understand that this principle applies to all matter. One astronomer, Ismaël Bullialdus, believed that the sun's gravity repelled objects that came too close, and attracted objects that got too far away, rather like a spring.

An interesting thing that most people overlook when they're watching a football flying through the air is that the force of gravity acts both ways. Gravity isn't acting on only the ball: it's also acting on Earth. In fact, the force that is acting on Earth is just as strong as the force acting on the ball.

You may wonder then why Earth doesn't move towards the ball when the ball moves towards Earth, but the truth is that it does. We just can't measure it because Earth has a very high mass and doesn't move as far as the ball does.

The equation I gave can be revised to reflect the effect of Earth's mass, m₁, and the mass of the football, m₂:

F = cm₁m₂/d²

So the heavier the football, the stronger the force acting on both Earth and the football. A football twice as large experiences twice the force. It doesn't fall twice as fast, though, because the force is spread out over twice as much mass. If Earth was twice as massive, the ball would fall twice as fast, because Earth would be pulling it twice as hard (assuming the ball had the same distance from Earth's center in both cases).

One interesting thing to note is that a heavier object does fall faster than a lighter object. This is because a more massive object pulls harder on Earth, causing Earth to move more quickly towards the object. The object therefore accelerates slightly faster relative to Earth's center. The effect is too slight to be measured with household items, but once you start working with planets it gets pretty obvious. A planet with Earth's mass will fall twice as fast as a football when placed at a given distance. Try it; you'll see!

One more thing is needed in the gravity equation: a value for the constant. I won't work it out here, but it can be found from Earth's acceleration of 9.8 m/s², mass of 5.974×10²⁴ kg, and radius of 6371 km. The value of the constant is 6.674×10^-11N·m²/kg². This constant is the same no matter what part of the universe you visit.

Now let's return to the problem I gave at the beginning. We have a universe filled with water. Let's use the common model that the universe has no bounds - that it's infinite. So we have an infinite amount of water. To make it easier, we can ignore effects due to the speed of light (because gravity waves move at the speed of light).

We must have infinitely high pressure then, right?

Let's see. To have pressure, the water must be pushing inwards. There must be a gravitational force. Is there? No. At every point, there are equal amounts of water in all directions. The force of gravity cancels itself out.

Think about the center of a sphere (like Earth). At a point outside the sphere, gravity pulls towards the sphere. But at the center of the sphere, gravity is pulling in every direction. The forces cancel each other out, and the net force is zero. It's like that with the water (except the sphere has an infinite radius, and every point is at the "center").

Without a gravitational force, there is nothing to cause a change in pressure. So nothing will happen.

Now what happens when there's a bubble? Well, there will be an area with no water, and no gravity. So the gravity will be pulling from every point, except for where the bubble is. As a result, the water around the bubble will be pulled by gravity away from the bubble, and the bubble will grow. We can assume that the bubble has the same pressure as the surrounding liquid (so it isn't a vacuum). As it grows larger, its rate of growth will accelerate (kind of like the universe's current expansion).

The water's surface tension will not resist this expansion, as far as I can tell, because an infinite amount of water is being affected by the gravity, but a finite amount of water is affected by the surface tension.

The compressibility of water, interestingly enough, does not come into play here. That can be found using fluid dynamics. The acceleration of the liquid can be worked out using basic physics. I'll leave that to you.

New posts every month - subscribe for free!